Newton’s Law of Cooling Formula, Experiment , Equation Derivation for Class 12

Newton’s Law of Cooling

The rate of heat loss of a body is directly proportional to the difference in temperatures between the body and its surroundings, according to Newton’s law of cooling. The law is typically qualified by the requirement that the temperature difference be modest and that the nature of the heat transfer mechanism remain unchanged. As a result, it’s the same as saying the heat transfer coefficient, which mediates heat losses and temperature changes, is constant.

Because the thermal conductivity of most materials is only slightly dependent on temperature, this requirement is generally met in heat conduction (where it is guaranteed by Fourier’s law). For forced air or pumped fluid cooling, when the fluid characteristics do not vary significantly with temperature, Newton’s Law is obeyed, but it is only essentially true for buoyancy-driven convection, where the velocity of the flow increases with temperature difference. Finally, Newton’s law of cooling only applies to very tiny temperature changes in the case of heat transfer by thermal radiation.

Newton’s Law of Cooling Formula

The heat transfer literature’s version of Newton’s law expresses the idea that a body’s rate of heat loss is proportional to the temperature differential between the body and its surroundings. The statement for a temperature-independent heat transfer coefficient is:

where Q is the rate of heat transfer out of the body (SI unit: watt), h is the heat transfer coefficient (assumed independent of T and averaged over the surface) (SI unit: W/m2K), A is the heat transfer surface area (SI unit: m2), T is the temperature of the object’s surface (SI unit: K), T env is the temperature of the environment (SI unit: K), T (t) = T (t) T (SI unit: K).

The heat transfer coefficient h depends upon physical properties of the fluid and the physical situation in which convection occurs. Therefore, a single usable heat transfer coefficient (one that does not vary significantly across the temperature-difference ranges covered during cooling and heating) must be derived or found experimentally for every system that is to be analyzed.

Formulas and correlations are available in many references to calculate heat transfer coefficients for typical configurations and fluids. For laminar flows, the heat transfer coefficient is usually smaller than in turbulent flows because turbulent flows have strong mixing within the boundary layer on the heat transfer surface. Note the heat transfer coefficient changes in a system when a transition from laminar to turbulent flow occurs.

Newton’s Law of Cooling Class 12 Experiment

When the internal thermal resistance within the object is small in comparison to the resistance to heat transfer away from the object’s surface (by external conduction or convection), which is the condition for which the Biot number is less than about 0.1, simple solutions for transient cooling of an object can be obtained. This condition allows for the assumption of a single, somewhat constant body temperature that varies with time but not with place. (Otherwise, the body would be at a variety of temperatures at any given time.) As time passes, the temperature of this single object will fluctuate exponentially (see below).

The so-called lumped capacitance model is created when the Biot number is low. The internal energy (the quantity of thermal energy in the body) is computed using this model, which assumes a constant heat capacity. In that instance, the body’s internal energy is a linear function of the single internal temperature of the body.

Following is a lumped capacitance solution that assumes a constant heat transfer coefficient, like in forced convection. The lumped capacitance model may be solved for free convection using a heat transfer coefficient that varies with temperature difference.

Newton’s law of cooling Equation Derivation

According to Newton’s law of cooling, we know that

dT/dt ∝ (T–Ts)

⇒dT/dt = −k(T–Ts)

The below zero or negative value on the Right side of the Equation indicates that the rate of temperature change decreases. If we suppose T_f is the temperature at time t and T₀ is the temperature in the start.

By Application of Integration on both sides, we get

_Tf∫^T0dT/(T–T_s) = ∫ (−k ∗ dt)

⇒ln((T_f–T_s)/(T₀–T_s)) = −kt

⇒(T_f–T_s)/(T₀–T_s) = e^−kt

⇒Tf–Ts=(T₀–T_s) e^−kt

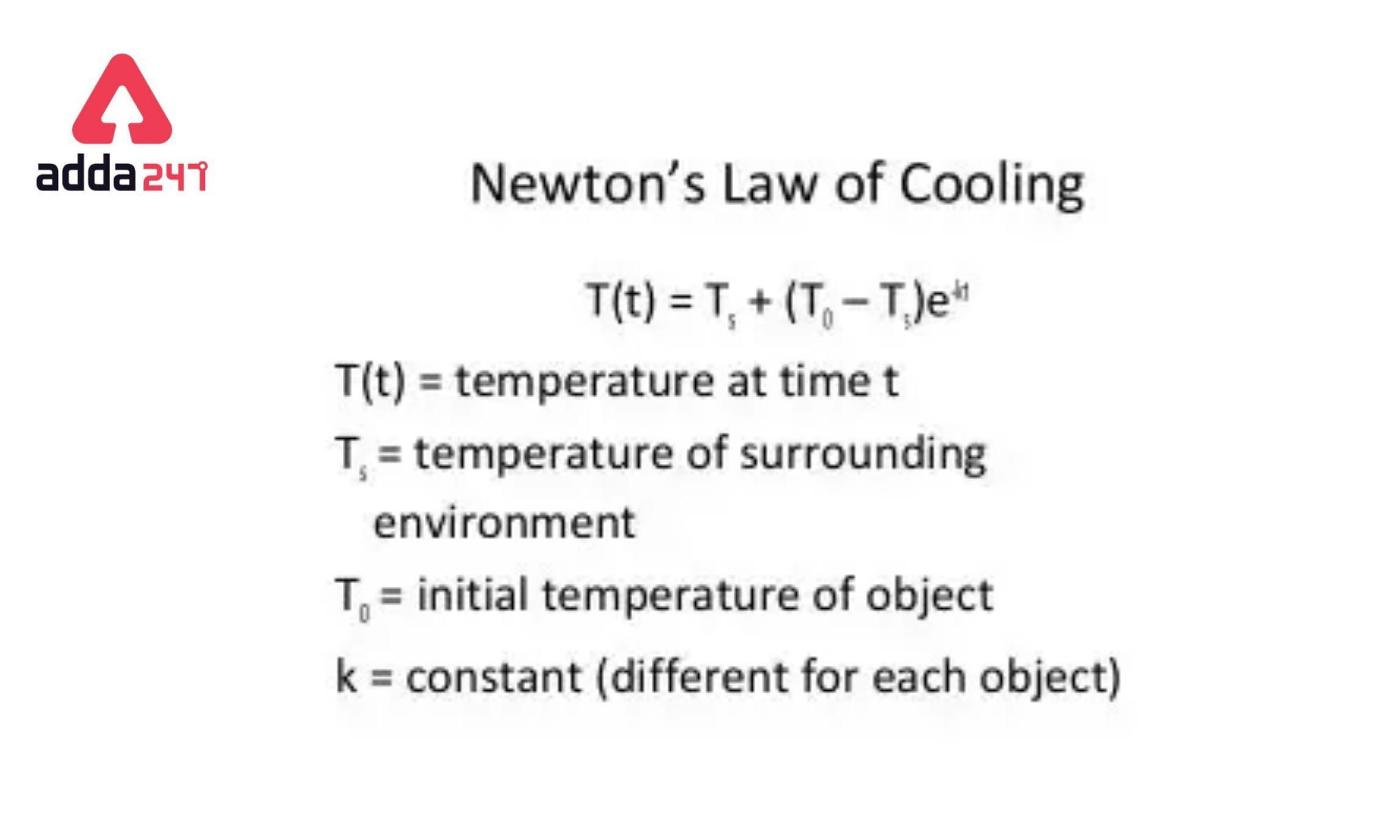
Therefore, T_f=T_s+(T0–Ts)e−kt

Hence,

Hence Proved

Newtons Law of Cooling- Limitations

Following are the limitations of the Newton’s Law of Cooling:

• The temperature difference between the body and the environment must be minimal.
• Only radiation should be used to lose heat from the body.
• The temperature of the surroundings must remain constant during the cooling of the body, which is a key constraint of Newton’s law of cooling.

Related Post:

• Kolbe Electrolysis Reaction- Definition, Mechanism Class 12
• Laws Of Motion By Newton- First, Second, Third, Applications
• Full Wave Rectifier- Formula, Working, Efficiency, Circuit
• Decomposition Reaction- Formula, Example For Class 10

Newton law of cooling QNAs

What is Newton’s law of cooling?

The pace at which an object cools is proportional to the temperature difference between the object and its surroundings, according to Newton’s law of cooling. Simply explained, in a cold room, a glass of hot water will cool down faster than in a hot room.

Define Newton’s law of cooling and warming?

The temperature of a body varies at a rate proportional to the difference in temperature between the body and its surroundings, according to Newton’s law of cooling (or heating).

What is Newton’s Law of Cooling how does it differ from Stefan’s law?

Stefan’s law can be used to calculate the temperature of a black body radiating heat. Only when the temperature differential between the body and the surrounding is modest and cooling happens due to response can Newton’s law be employed. There should also be no heat loss owing to conduction.

What is a cooling curve in chemistry?

A cooling curve of a substance is a graph of the temperature variation over time as the substance is allowed to cool. The gradient of the cooling curve is determined by the heat capacity of the substance, its thermal conductivity, and the ambient temperature.

What is meant by a black body?

A blackbody is a physics term for a surface that absorbs all radiant radiation falling on it. The word comes from the fact that incident visible light is absorbed rather than reflected, making the surface appear black.

Sharing is caring!