How do you calculate a factorial?

To find the factorial of a number, multiply it by the previous number's factorial value. The product of all positive integers less than or equal to n is the factorial of a non-negative integer n, denoted by n! in mathematics. The product of n with the next smaller factorial is also equal to the factorial of n: As an example, According to the convention for an empty product, the value of 0! is 1.

Why do we use factorials?

You might be wondering why the factorial function is important to us. It comes in handy when we're trying to count how many various ways we can combine things or how many possible orders there are for things. How many different ways can we arrange n things, for example? For the first thing, we have n options.

How many zeros are there in the 100 factorial?

there are 24 zero in factorial of hundred.

What number is 9.332622 E 157?

The number 9.332622 E 157 is 100 Factorial.

How do you calculate a factorial?

In short, a factorial is a function that multiplies a number by every number below it till 1. For example, the factorial of 4 represents the multiplication of numbers 4,3, 2, 1, i.e. 3! = 4×3 × 2 × 1 and is equal to 24.

What is the Factorial of 100?- Check in Voice Command -

Q: What is the factorial of 10?

What is a ten-fold factorial. The factorial of 10 has a value of 3628800, i.e. 10! = 10 9 8 7 6 5 4 3 2 1 = 3628800.

Table of Contents

The factorial of 100, denoted as 100!, is a massive number representing the product of all positive integers from 1 to 100. Factorials, commonly used in fields like mathematics, physics, and computer science, are crucial for calculating permutations, combinations, and probability. As numbers grow, factorial values increase at an astonishing rate, making even 100! a challenging calculation. Understanding and computing large factorials like 100! require efficient algorithms and powerful computational tools, as standard calculators cannot handle the magnitude of such values. This article delves into the significance of factorials, methods of computation, and applications in various scientific fields. Check here What is the Factorial of 100, in number, in voice command.

What is the Factorial of 100?

9.332622e+157

After calculation, the value of Factorial 100 comes out to be equal to 9.332622e+157.

What is the factorial of 100 voice command?

To know what is the factorial of 100 voice command press the play button:

What is the factorial of 100 speak?

The factorial of 100, written as
100
!
100!, is an extremely large number:

100
!
=
93
,
326
,
215
,
443
,
944
,
152
,
681
,
699
,
238
,
856
,
266
,
700
,
490
,
715
,
968
,
264
,
381
,
621
,
468
,
592
,
963
,
895
,
217
,
599
,
993
,
229
,
915
,
608
,
941
,
463
,
976
,
156
,
518
,
286
,
253
,
697
,
920
,
827
,
223
,
758
,
251
,
185
,
210
,
916
,
864
,
000
,
000
,
000
,
000
,
000
,
000
,
000
100!=93,326,215,443,944,152,681,699,238,856,266,700,490,715,968,264,381,621,468,592,963,895,217,599,993,229,915,608,941,463,976,156,518,286,253,697,920,827,223,758,251,185,210,916,864,000,000,000,000,000,000,000
In words, this would be spoken as:

“Ninety-three quattuordecillion, three hundred twenty-six tredecillion, two hundred fifteen duodecillion, four hundred forty-three undecillion, nine hundred forty-four decillion, one hundred fifty-two nonillion, six hundred eighty-one octillion, six hundred ninety-nine septillion, two hundred thirty-eight sextillion, eight hundred fifty-six quintillion, two hundred sixty-six quadrillion, seven hundred trillion, four hundred ninety billion, seven hundred fifteen million, nine hundred sixty-eight thousand, two hundred sixty-four”

… and so on.

Because it’s so large, we often just refer to it in scientific notation: 100!≈9.3326×10 to power 157

This is a more practical way to communicate the value.

How to calculate What is the factorial of Hundred(100)?

The answer of what is the factorial of 100

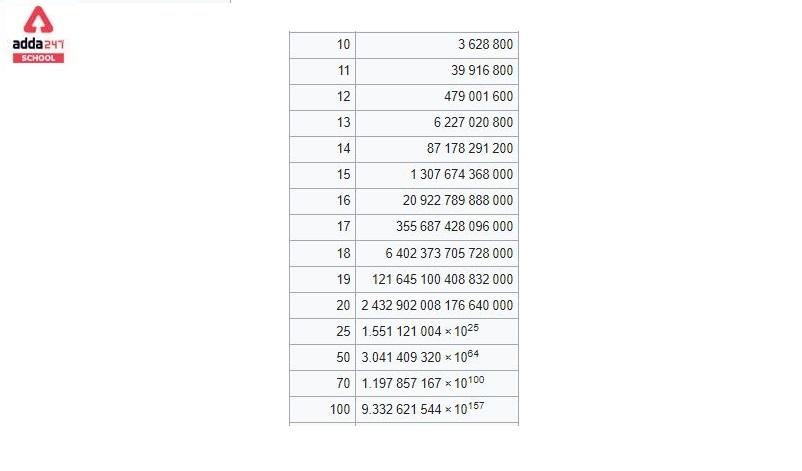
- 100! is exactly: 93326215443944152681699238856266700490715968264381621468592963895217599993229915608941463976156518286253697920827223758251185210916864000000000000000000000000
- The approximate value of 100! is 9.3326215443944E+157.
- The number of trailing zeros in 100! is 24.
- The number of digits in 100 factorial is 158.
- The factorial of 100 is calculated, through its definition, this way:

100! = 100 • 99 • 98 • 97 • 96 … 3 • 2 • 1

What is Factorial?

The product of all positive integers less than or equal to a given positive integer, indicated by that integer plus an exclamation point, is known as actorial in mathematics. As a result, factorial seven is expressed as 7!, which means 1 2 3 4 5 6 7. The factororial zero is equal to one. In the evaluation of permutations and combinations, as well as the coefficients of terms in binomial expansions, factororials are frequently encountered Nonintegral values have been included in factororials. Factorials were found by Jewish mystics in the Talmudic book Sefer Yetzirah and by Indian mathematicians in the canonical works of Jain literature. The factorial operation appears in many fields of mathematics, particularly in combinatorics, where its most fundamental use counts the number of unique sequences – permutations – of n distinct objects: there are n! Factorials are employed in power series for the exponential function and other functions in mathematical analysis, and they can also be found in algebra, number theory, probability theory, and computer science.

Much of the factorial function’s mathematics was established in the late 18th and early 19th century. Stirling’s approximation approximates the factorial of huge numbers accurately, demonstrating that it grows faster than exponential growth. Legendre’s formula can be used to count the factorials’ trailing zeros by describing the exponents of prime numbers in a prime factorization of the factorials. The factorial function was interpolated by Daniel Bernoulli and Leonhard Euler to a continuous function of complex numbers, the gamma function, except for the negative integers. The factorials are closely related to many other famous functions and number sequences, including binomial coefficients, double factorials, falling factorials, primorials, and subfactorials. Implementations of the factorial function are used in scientific calculators and scientific computing software libraries and are frequently cited as examples of various computer programming approaches. Although computing big factorials directly using the product formula or recurrence is inefficient, faster algorithms exist that match the time for fast multiplication procedures for numbers with the same number of digits to within a constant factor.

What is Factorial of 100- How to Calculate Factorial?

The factorial function of a positive integer n n is defined by the product:

$What is the Factorial of 100?- Check in Voice Command -_3.1$

In product notation, this might be stated more succinctly as

$What is the Factorial of 100?- Check in Voice Command -_4.1$

If all but the last term is kept in this product formula, it will define a product of the same form for a smaller factorial. This results in a recurrence relation, according to which each factorial function value may be generated by multiplying the preceding value by n.

$What is the Factorial of 100?- Check in Voice Command -_5.1$

Factorial of 100- Applications of Factorial

The factorial function was first used to count permutations: there are n! different ways to arrange n different objects into a series. Factorials are used more widely in combinatorics formulas to account for different object orderings. The binomial coefficients (n k), for example, count the k-element combinations (subsets of k elements) from a collection of n elements and can be obtained using factorials. The factorials are added to the Stirling numbers of the first kind, and the permutations of n are counted in subsets with the same number of cycles. Counting derangements, or permutations that do not leave any element in its original location, is another combinatorial application; the number of derangements of n items equals the nearest integer to n! / e.
The binomial theorem, which employs binomial coefficients to expand powers of sums, gives rise to factorials in mathematics. They can also be found in the coefficients that are used to connect specific families of polynomials, such as Newton’s identities for symmetric polynomials. The factorials are the orders of finite symmetric groups, which explains their application in counting permutations algebraically. Factorials appear in Faà di Bruno’s formula for chaining higher derivatives in calculus. Factorials regularly appear in the denominators of power series in mathematical analysis, most notably in the series for the exponential function.